Hi, my name is Justin Kulp…
… and I am a Research Assistant Professor at the Simons Center for Geometry and Physics and the Yang Institute for Theoretical Physics in Stony Brook, New York. This site is a place for me to keep a record of my projects and research. I hope it will act as a sort of virtual academic scrapbook.
From 2019-2023 I was a PhD student at the Perimeter Institute for Theoretical Physics and the University of Waterloo. My supervisors were Davide Gaiotto and Jaume Gomis. I graduated from Perimeter Scholars International at the Perimeter Institute in the 2018/2019 cohort. Before that, I graduated with an HBSc. in Mathematics and a minor in Physics from Lakehead University in 2017. My CV and some more detailed accounts of my research can be found on this website.
My current research focuses on studying quantum field theory as a subject in its own right: mostly from a high energy theory perspective, but there is overlap with mathematical physics and condensed matter physics. I also have a passing interest in particle phenomenology. In particular, I have been focusing on topological aspects of quantum field theories, anomalies, and defects, especially in the context of TFT and CFT. My publications and public presentations are available on this site.
When I’m not pretending to be a physicist or mathematician, I can be found playing guitar or at the gym. The icon for this site is a “thick” Penrose rhomb that has been inflated using the associated inflation rules for a few iterations.
What I’m Doing: Current Research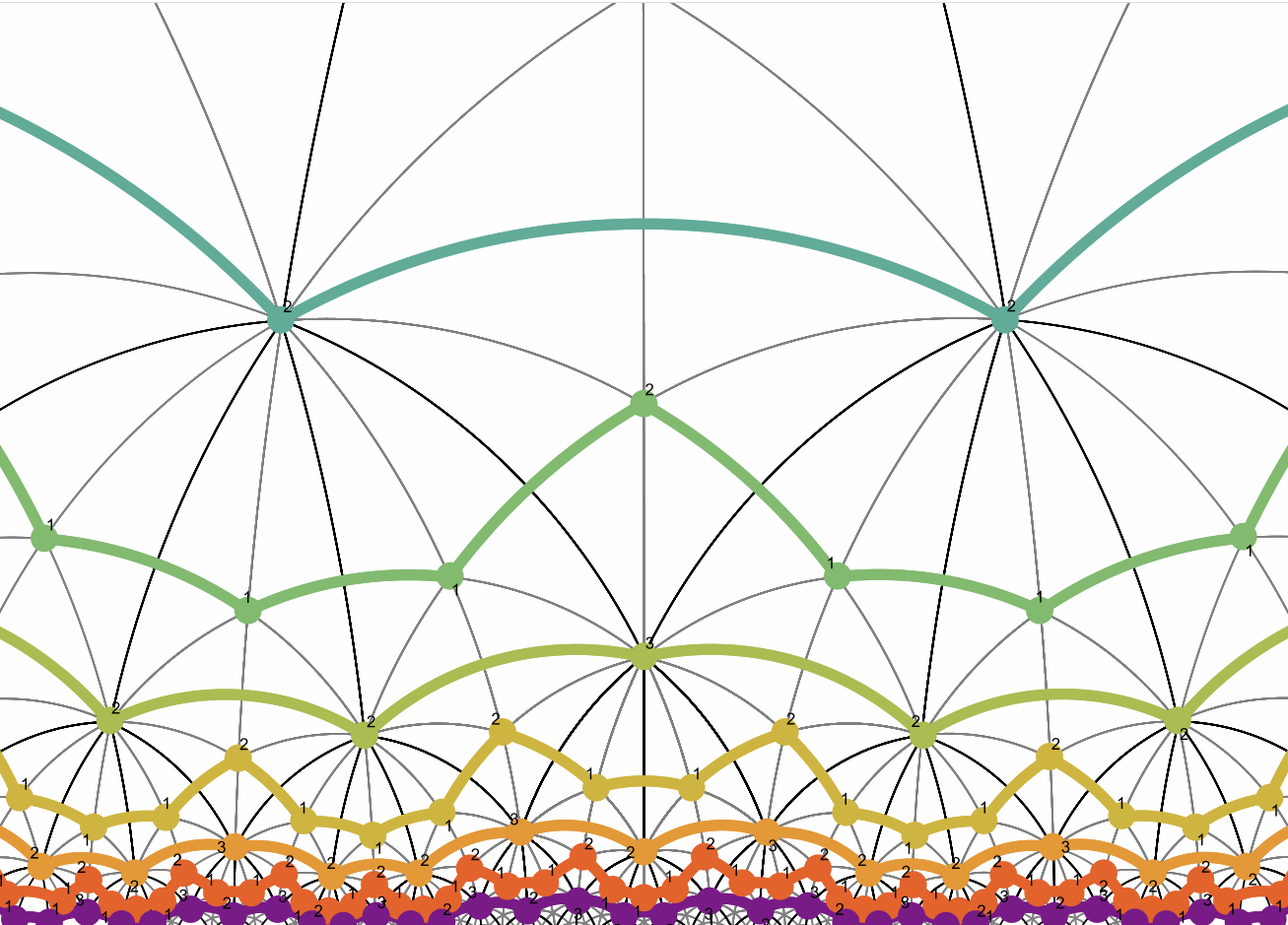
I’m currently working on projects studying:
- Global categorical symmetries
- Twisted quantum field theories
In the picture is the {3,7}-tiling of the hyperbolic plane (in black) and its dual tiling (in grey). Strips of the tiling are coloured like a rainbow for fun, see the paper here.
What I’ve Done: Past Research
Recently, I’ve worked on projects on
- Aperiodic tilings and their application to physics
- Making celestial holography semi-rigorous
- Topological manipulations of QFTs
- Twists of SUSY QFTs
- Crossing symmetry in Chern-Simons Matter theories